How To Calculate Standard Deviation?

Data is normally dispersed with no distortion in regular dispersals. Most outcomes are consolidated in a prominent spot, with the diminishing value as it goes away from the base. The standard deviation demonstrates to what extent your adherence is on the median from the allocation centre.

Numerous technical variables, like altitude, regularised test numbers, and the employment fulfilment rating value, have regular distributions. And when you have already understood the standard deviations of numerous samples, you might use statistical trials to analogise the dispersals and identify the deductions around the larger populations.

Consider the standard deviation as an amount of variation in your collection on a moderate range . It discloses to you how far per number differs from the mean on the average. Variables remote from the mean have an elevated standard deviation, whereas those who are near the mean have a lower standard deviation.

What Do You Understand by Normal Deviation?

As we have already mentioned, data is normally scattered with zero bias in a normal regular distribution. Most values are focused in a mid zone and diminishing as they go out from the centre. In this type of distribution, the variables of central i.e, mean, mode, and median are similar.

The Empirical Rule of Standard Deviation

If your distribution follows a normal distribution, the standard deviation & mean can tell you where most of the numbers are located.

The empirical rule, often comprende das the rule of 68-95-99.7, indicates where your values are located–Around 68 per cent of scores are within the single standard deviation of the mean, 95 percent of which are within the double standard deviations from the mean, and 99.7 percent are under the three standard deviations from the mean.

The empirical rule is a simple technique to rapidly review your data and look for anomalies or extreme results that don't fit the trend.

The standard deviation is a somewhat accurate measure of variability for non-normal distributions and should be used in conjunction with other measures such as the range or quartiles range.

Here are the Steps For Determining the Standard Deviation

The standard deviation is generally determined automatically by the statistical analysis programme you're using. You may also do it by hand to better understand how the formula operates. The standard deviation may be calculated by hand in six steps. We'll utilise a tiny data set of six scores to proceed through the processes.

Number 1: Identify the mean

Calculating the mean requires two steps: adding all attribute values set and dividing that number by the possible values.

Number 2: Identify the score deviation from each mean

To determine the value from each mean, you have to deduct the mean from each score.

Number 3: Each standard deviation from the mean is squared.

In the next step, each variation from the mean is multiplied by itself. Positive numbers will arise as a result of this.

Number 4: Calculate the aggregate of squares.

Now calculate and make the addition of all the squared deviations together. The process is commonly referred to as the sum of squares.

Number 5: Determine the difference.

The variance is calculated by dividing the sum of the square by n – 1 (for a sampling) or N (for a sample).

Number 6: Calculate the variant's square root.

The standard deviation is calculated by taking the square root of the dispersion.

Learn how to acknowledge any source of information using the APA 7th style.

Download Now

What Makes the Standard Deviation Such an Effective Measure of Variability?

Although there are more effortless approaches to identify the value of the variation, the standard deviation equation favours unevenly distributed samples over uniformly distributed samples. A greater standard deviation suggests that the dispersion is more comprehensive and more unequally disseminated. This indicates that it delivers a more precise picture of the variability in your data than more straightforward proportions like the difference in mean absolute.

The Mean Absolute Deviation is analogous to the standard deviation though it is more straightforward to calculate. First, turn each divergence from the mean into favourable integers and portray them as conclusive values for example, -5 turn into 5.

The Mean Absolute Deviation, unlike standard deviation, does not need the calculation of squares or square roots of values. And as an outcome, it furnishes you with a less exact estimation of the variable.

Tips to consider while determining the standard deviation

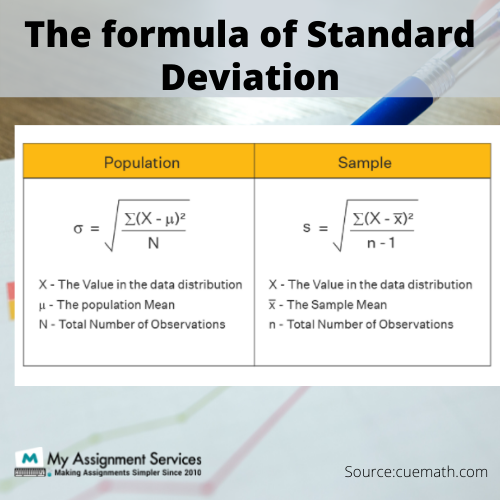
• The standard deviation is the square root of the aggregate squared deviations of data readings from the mean with n as the sample or population size.
• The increasing square root of the variable is the standard deviation.
• The standard deviation measures the distribution of data ends around the mean.

What is Sample Standard Deviation in Statistics?

Because sampling every community member is not always practicable, the preceding equation must be changed to calculate the standard deviation using a randomised sample of the population under study. The standard deviation, usually indicated by s, is a frequent estimate. It's worth mentioning that there are a plethora of equations for computing sample standard deviation since, except for the sampling mean, sample standard deviation lacks a single unbiased, efficient, and maximum probability estimator.

It's a revised version of the equation that eliminates some of the distortions in the equation by altering the population standard deviation calculation by utilising the sample size as the population size. On the other hand, the unbiased standard deviation estimate is time-consuming and varies based on the distribution. As a result, the "revised sample standard deviation," often known as the "sample standard deviation," is the most widely used estimate for population standard deviation.

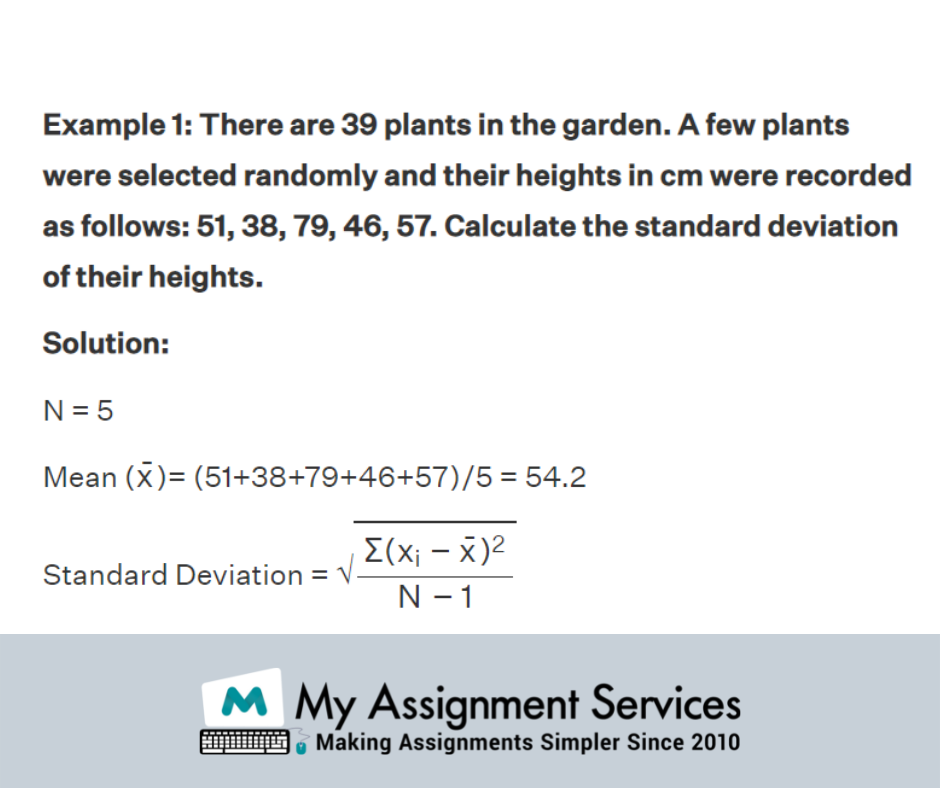
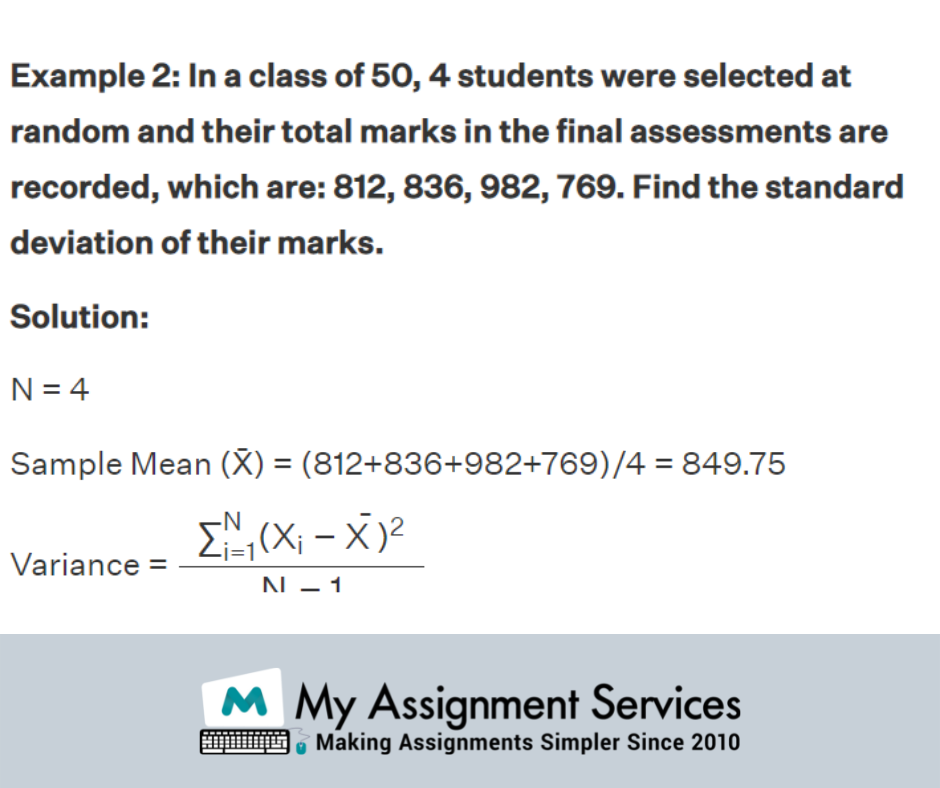
Some examples of standard deviation

How can our experts assist you in both experimental and industrial contexts, the standard deviation is commonly used to verify theories against real-world data. Quality assurance for some commodities is an illustration of this in commercial processes. Standard deviation could be used to determine a measured value within which a product feature should fall a large majority of the time. To guarantee quality control, it may be required to make adjustments to production if values go beyond the calculated range.

You can get away with standard deviation if you are willing to pursue your career in studying. To command all the formulas, you need proper guidance apart from the practice and deductions. Our experts are subject matter specialists having vast experience in and knowledge of statistics and can assist you with any statistics assignment help. You can connect with them via one-on-one guided learning sessions and explore much more about statistics other than standard deviations.